When using the Huygens MTF analysis, it’s important to know how the different settings impact your output data – specifically, the Pupil Sampling, Image Sampling, and Image Delta values. To illustrate this, let’s take a look at the “Wide angle lens 100 degree field” sample file (the default location for this is at “C:\ … \Zemax\Samples\Sequential\Objectives”).

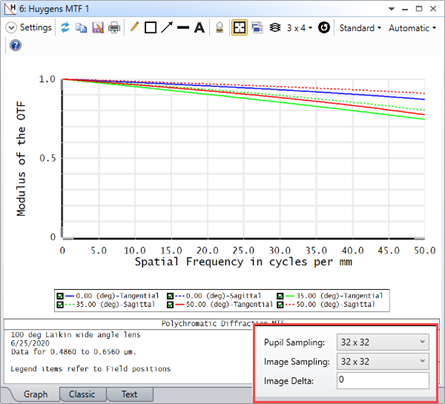
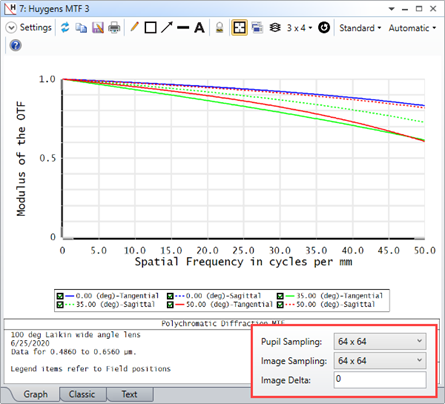
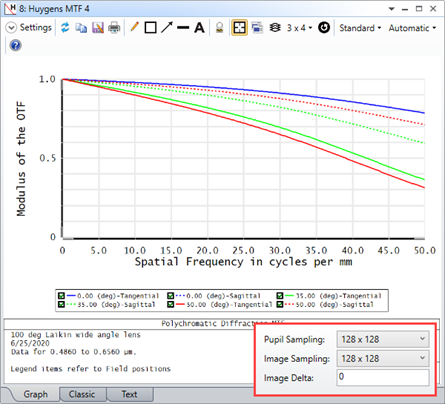
While it seems like the analysis is generating pretty different results, there also needs to be some consideration to how the results in the Huygens PSF look with these same settings, as they are identical to the ones in the Huygens PSF analysis. There, we can see that we are in fact undersampling the PSF by not having a wide enough array, which breaks one of the requirements in the validity of the Huygens MTF analysis.

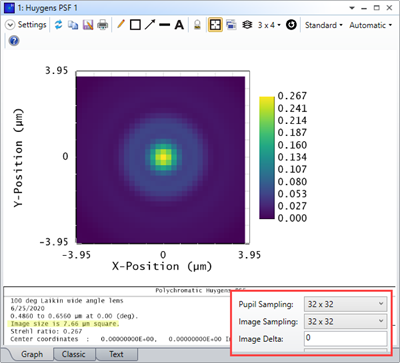
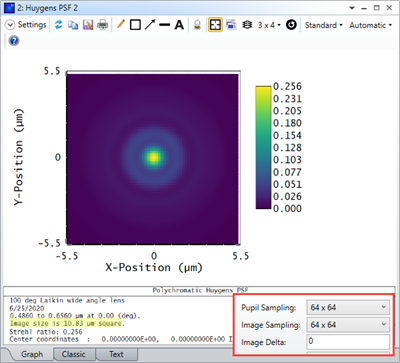
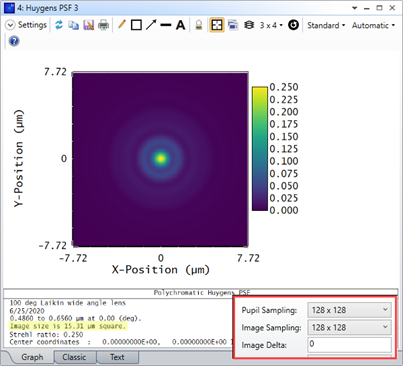
Adjusting the physical size of the image of the PSF is related to the Image Sampling (how many points we take at the image plane) and Image Delta (the physical pixel-to-pixel spacing in terms of microns) values. In this case, with an Image Delta value of 0 – which computes a default image delta based on the working F/# of your system and the primary wavelength – we can see that we are not adequately observing the entire PSF. Therefore, one approach we can take is to ensure that the Image Delta sampling is set between each Image Sampling computation to observe the full PSF across our Field of View. For instance, at an Image Sampling of 128x128, we can have an Image Delta of 0.4 microns.

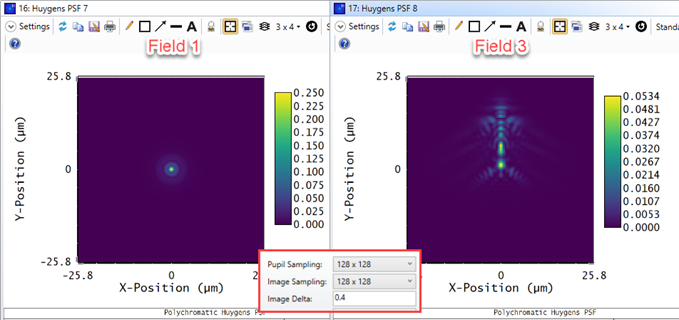
With this in mind, and by adjusting the Image Delta across the different sampling settings in this analysis to maintain the same PSF image size, we get improved convergence in our Huygens MTF analyses:

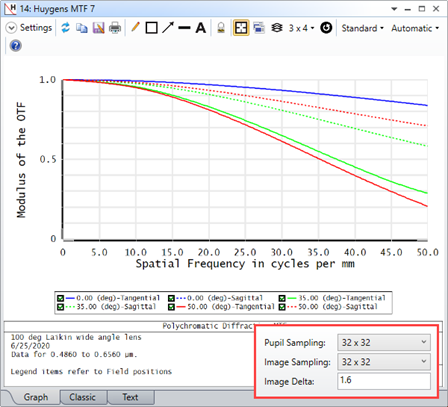
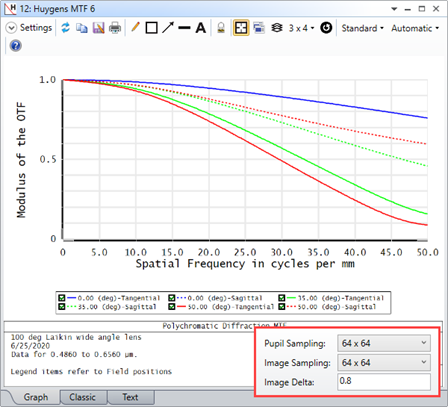
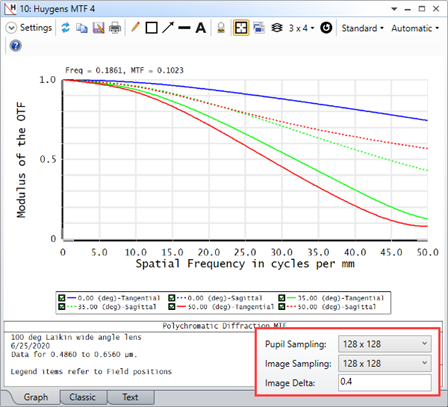
That said, it’s also good to note when the Huygens MTF result will be most useful. In this sample file, if we take a look at the Spot Diagram and display the Airy Disk, we can see the file at this stage is not diffraction-limited across our field of view:

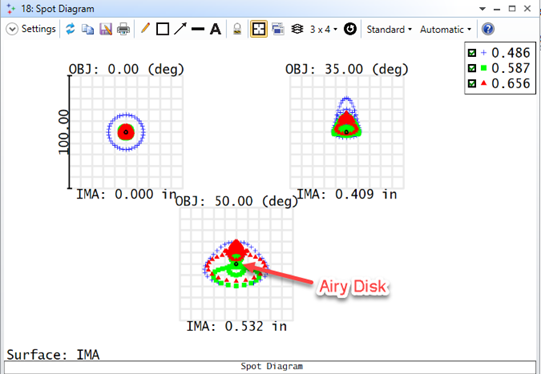
Since our system’s performance will be much more dominated by aberrations rather than the diffraction effects at the image plane, you can leverage the Geometric MTF results at this stage to give an approximate MTF which agrees well with the Huygens results in significantly less computation time:

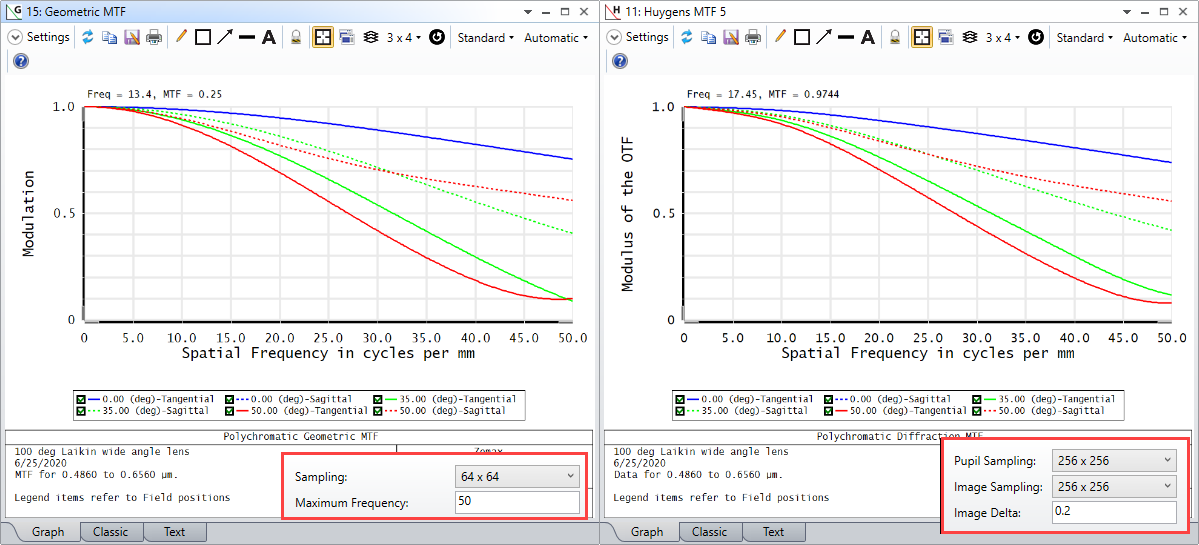
So, in short, you always want to keep in mind how well-corrected your system is so that you can select the analyses that are most relevant for your model. Furthermore, when using the Huygens MTF results, it’s key to consider that your settings are defined such that they adequately sample the PSF for best, most accurate results.