This question comes up often. Siegman’s M^2 term measures the deviation of a Gaussian beam from the ideal case. It is vital to recognize that the M^2 is a measure, not a parameter. Think of it as analogous to the standard deviation of a data set. There are unlimited ways to get the same measure. For a Gaussian Beam, scaling the beam size and divergence values by the value of M will produce a beam quality factor of M^2, but this is merely one way to do this. The Paraxial Gaussian Beam tool does not have many degrees of freedom, so this makes sense, but it would be a disservice to have a generic M^2 parameter for POP beams because it can mean anything.

The issue can be easily demonstrated by looking at a couple ways to generate the same M^2 from a starting Gaussian Beam in POP. The attachment to this post contains an arbitrary sample system and a POP tool. Initially, the beam is slightly focused and brought to an image surface well before focus.

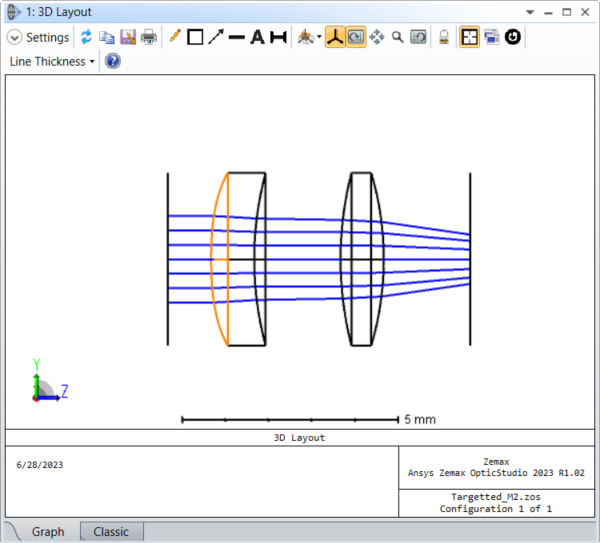
We can begin by making the fourth and sixth order terms for the Even and Odd Aspheres variable, for a total of four variables. A look at the Merit Function Editor shows that we are starting with a quality factor of 12.8 and are trying to create a beam with a factor of 20. The POPD operands impose this target measured in X and Y.

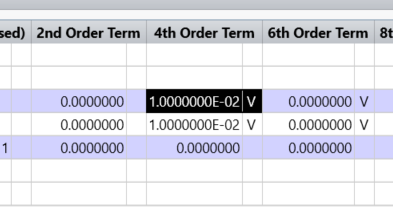
The initial beam is essentially Gaussian for all appearances.

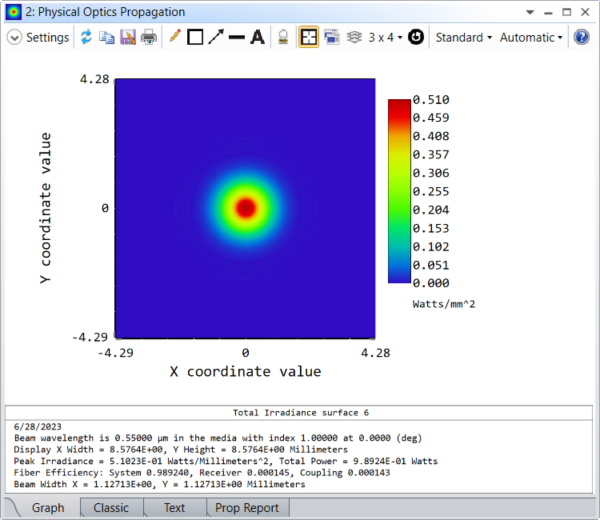
In a single cycle, the beam is shaped to an M^2 of 20 by shaping it to diverge strongly, creating a larger beam size as we can see:

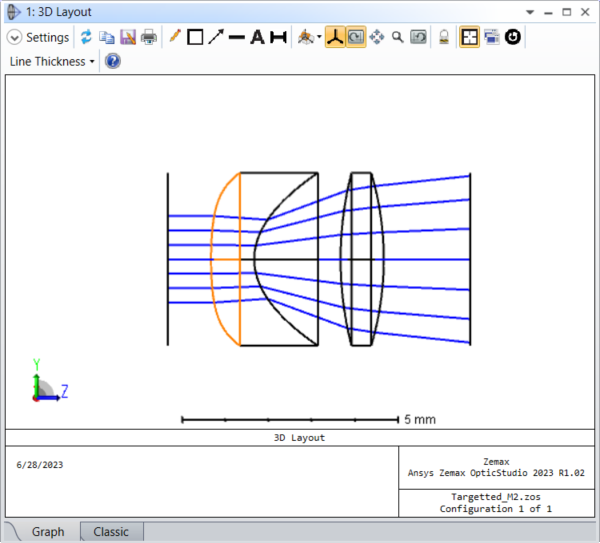
Undo these changes and remove the variable solves on the Aspheres. Now we can go to the Zernike Standard Sag surface and designate instead the terms Zernike 7 to Zernike 10 (parameters 21 to 24) as variable. Running the optimizer again to create a targeted quality factor of 20 yields these very different systems and beams:

The second shaped beam, with a quality factor of 20, is now converging and not even radially symmetric.

This method of generating beams with a specific quality factor is not the ideal method. Even using the same variables, a different set of initial conditions could lead the optimization to produce a different result. The better way to get a beam with a specific value is to ask what about your initial beam is causing the deviation from the perfect Gaussian beam. If you have a source that comes from a manufacturer, you may be able to get a beam file (.ZBF) with the initial beam stored and ready to go. Options like the DLL are also available for users that wish to specify the beam pixel by pixel.

For a quick-and-dirty initial beam with a specific M^2, this field shaping approach may be viable, but remember always that you are not creating a unique beam in this way.

Great answer Kevin. This question is similar to ‘how to create a wavefront with lamdba/2 error’ or whatever error. You can, of course, create an infinite number of such wavefronts.

The real problem is that the user has a measured beam with a Gaussian width and some non-unit M^2. To better characterize the beam, we need measurements of the beam at multiple distances of propagation, and specifically some interferograms of the beam so that we have phase information as well as beam size.

The good news is that as M^2 increases you can just use rays. Look at the propagation distances you are interested in, and compare them to the Rayleigh range. If the RR is small compared to the propagation distance, just use rays with a divergence angle equal to the M^2-scaled Gaussian beam divergence.

If you are working within the RR, then you need the information at multiple propagation distances. If you have an interferogram of the beam, then you can create the complex amplitude directly as you have intensity and phase data.

• Mark

While none of the arguments that M^2 is not uniquely descriptive, I would not agree that it is therefore pointless to try (or need) to create beams with M^2  slightly greater than unity.  The laser application industry is very particular about M^2 in this regime.  Measured M^2 < 2 nearly always divide the M between divergence and waist size and that is a working parameter that application engineers use to design real systems.  Customers routinely want to know how a system performs with a M2 1.3 laser.    It would be very useful if the POP tool Gaussian Waist + Angle were really what it sounds like, where you can control each dimension into the M2 you want.  Sure, you can get M2 many ways but these are the very 2 parameters that describe real lasers with M2 < 2.  

-Jay

Gaussian Waist and Angle is precisely what it sounds like...just enter the values of each scaled by sqrt(m^2) if you want to split the difference between the two. Or have you had some problem with doing that?

• M

As was already pointed out here, saying M^2 of 1.3 is incredibly ambiguous.  However, if you have to design systems with a specific M^2 value, I would check out Jeff’s post from a few months ago (still one of the more impressive uses of multiple different OpticStudio features):

Mark, that’s what I understood the Gaussian Size/Angle to mean until I used it.  At this writing that setting always produces a beam with M^2 = 1. It does afterall say Gaussian Size and not Waist Size.  POP will return a waist smaller than the waist that is input in this setting, and moves the waist appropriately to match the that is input.  I don’ t know, perhaps generating the field for this scenario is more difficult than it appears.

While I couldn’t agree more, Michael, that M^2 is an extraordinarly blunt measure, laser sales are won and lost on this merit for laser  M^2 < ~1.5.  In as much as the Zemax Peraxial Gaussian tool splits Div and W0 by \|M^2, I really wish that the POP Size/Angle did this because this is how nearly all commercial lasers perform.  It’s imperfect, but at least answer the question the customer is asking.  

Cheers.

Jay