The "paraxial lens" cannot be used for coherent calculations because the phase information is incorrect. The following help files are explained:

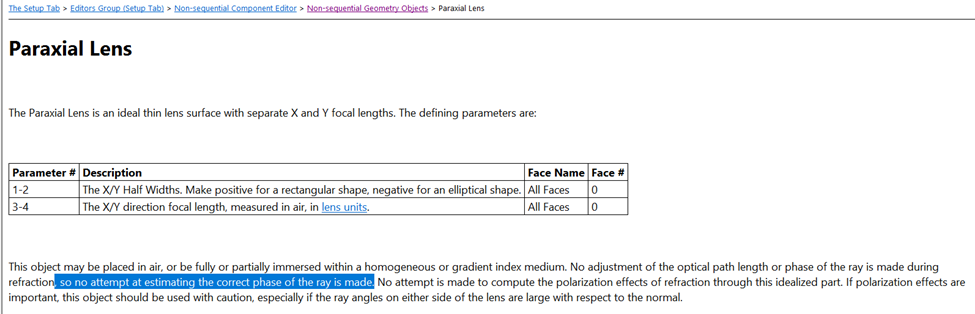
Please also refer to the following articles.

• Non-Sequential Paraxial Lens scrambles coherent phase

There are two workarounds:

(1) There is a simple workaround called Sweatt lens. Make a model material with a really high refractive index, e.g. 10001 and give the radius to achieve the desired focal length. OPD can be calculated for this real lens (in imaginary glass).

(2) In the article below, a user-defined "NSC_Paraxial_Lens.dll" provided.

This DLL can correctly calculate phase information and can be used for coherent calculations.

https://support.zemax.com/hc/en-us/articles/4403037789203-How-to-simulate-exit-pupil-expander-EPE-with-diffractive-optics-for-augmented-reality-AR-system-in-OpticStudio-part-4

I would really like an explanation from the Zemax development team as to why the NSC paraxial lens fails to track OPL phase.

Mike

I did not know the high-index method was called a Sweatt model. This is good to know now.

Hi Mike, do you think the information in this forum post helps?

When using Paraxial surfaces, why will the PLEN/OPTH operands return optical path results that are smaller than the path length? | Zemax Community

The answer to Mike’s question on the inability of NSC paraxial lenses to account for phase is a good one, and it has been troubling me for a while.

NSC is 100% free of paraxial approximations, and is based on real ray tracing only. So, there is no paraxial approximation for use as a reference, fro any kind of system. The NSC paraxial lens surface then is just an idealized surface that bends rays with a power given by the equation in the Help file.

But it’s more complex than that. In sequential mode, rays always arrive at the paraxial surface from the ‘object’ side and refract into the image side. Rays also come only from one point source per field and wavelength. That means you can establish a relationship between a ray landing somewhere in the pupil and a differential ray that is very close to it.

In NS mode, rays can come from any number of sources, and the paraxial lens can be illuminated from either side. Indeed, rays almost tangential to the paraxial surface will be refracted too. Rays can be split or scattered before they hit the NS paraxial. You cannot assume that all rays hitting the paraxial lens have originated from the same point, so you cannot define that type of phase relationship between them.

We thought about defining an ‘Imaging Group’ in NS mode that would define a single source, multiple objects, and a single detector. But, that’s really what sequential mode is for!

In retrospect we should not have added the paraxial NS lens at all, but instead a ‘camera module’ where you specify f/#, entrance aperture and detector size, and then just formed an image using the NS Paraxial equations and just not offered any phase information. But we never did :-(