Hi,
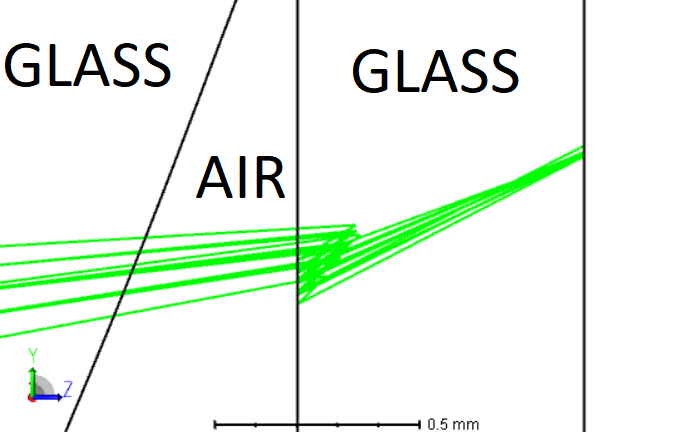
Got some strange result of ray reflecting from nothing.
At the ray trace window it mark this path length as negetive.
Moreover, the 3d layout dont allow ray fletch.
What went wrong?
Thanks,
Nadav
Hi,
Got some strange result of ray reflecting from nothing.
At the ray trace window it mark this path length as negetive.
Moreover, the 3d layout dont allow ray fletch.
What went wrong?
Thanks,
Nadav

Hi Nadav,
Are you in Sequential or Non-Sequential Mode?
If you are in Non-Sequential, this looks like it may be a nesting rule issue or a problem with the 'inside of' parameter on the source.
For the nesting rule, if two objects are overlapping each other, the rays will propagate according to the material properties of the object listed lower on the Non-Sequential Component Editor. For the 'inside of; parameter, if your source starts inside of an object, you will need to put the object number in the 'inside of' column of that source.
Are you able to send us the file so we can investigate further?
Hi Kaleb,
I am in Sequential mode. Can this be from aspheric terms of the first glass coupled with a large field angle?
Thanks for the answer,
Nadav
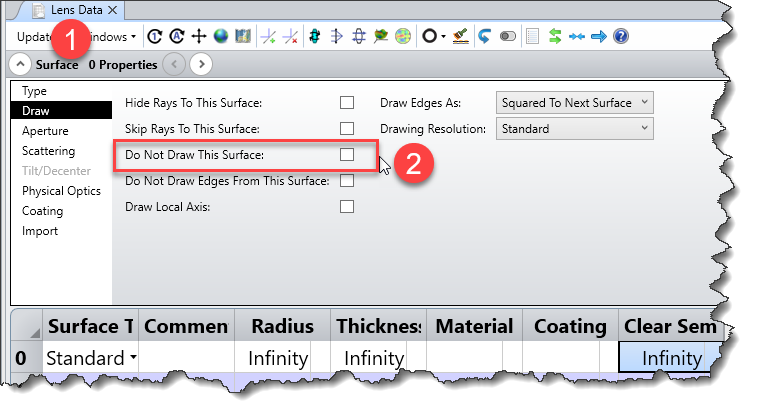
Check you do not have 'Do not draw surface' selected for any surface:
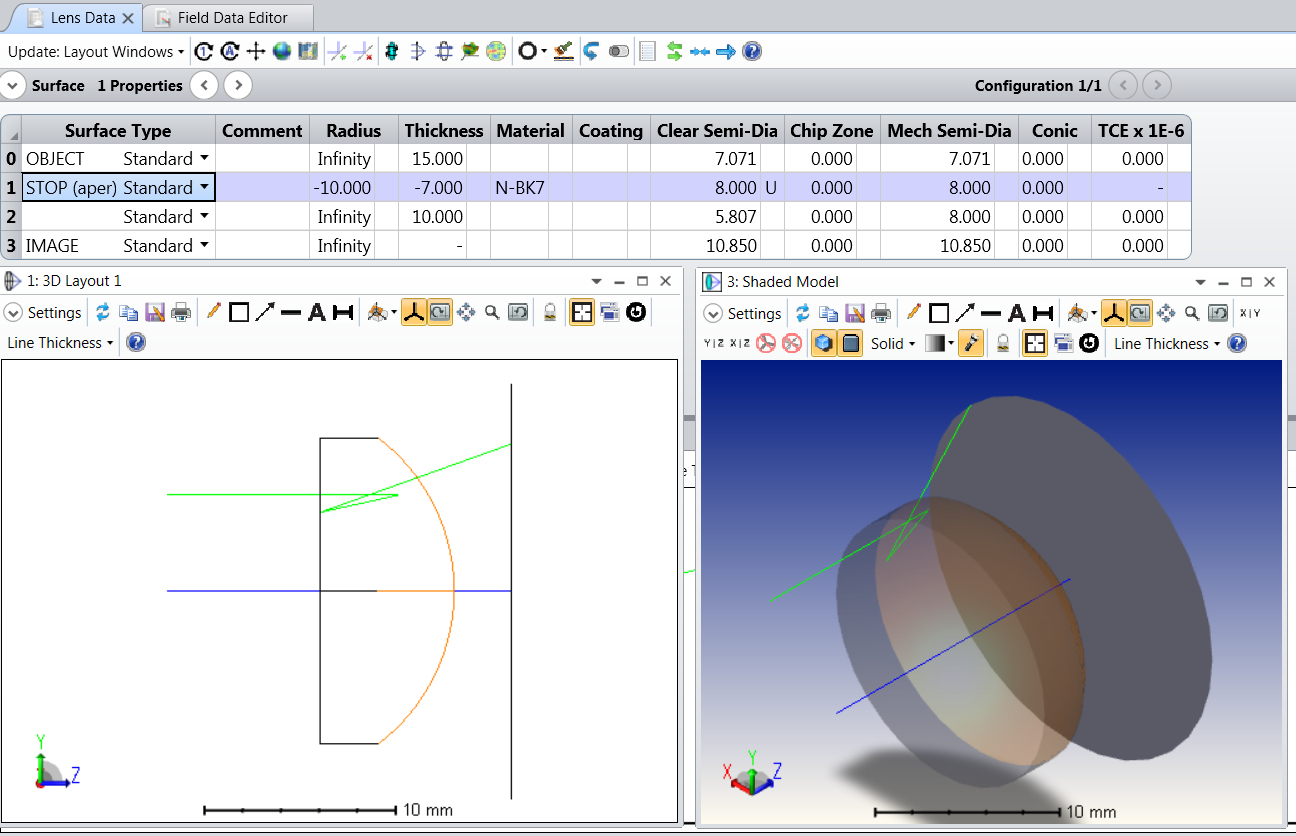
It's possible to generate this kind of confusion with a negative thickness. (Usually used for mirror spaces.) If that's the case, and you look at a complete 3D view, you will see the ray is reflected from a point on the surface which is not in the plane of the slice. (I also set hide lens edges on the layout.)
Hi all,
Mark - thanks for the tip, it was unchecked.
David - so it's also possible with extremely aspheric surfce (like my case)?
Thanks for the help,
Nadav
Hi Nadav,
With my example, the issue is the negative thickness in the LDE. If you don't have a negative thickess, then my last post does not help.
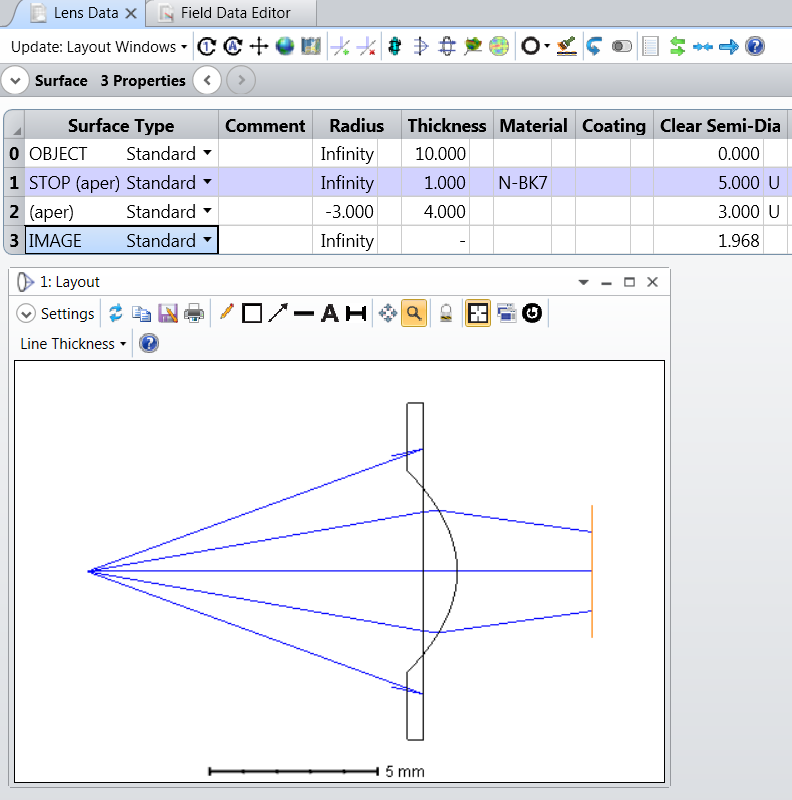
However, it is possible to create non-physical lenses where a region of the lens seems to have a negative thickness. In the example below, the curvatures and thicknesses are such as to produce such a case. Note the appearance of something that looks like a reflection. If you use MNEG in the merit function you will find the edge thicknss of surface 1 is negative. This uses a spherical surface -- it would be even easier to create such a problem using aspherics. You can avoid this by including a minimum edge thickness operand MNEG in the merit function or by using an edge thickness solve on the offending surface.
Okay, I think I understand now.
Thank for the help,
Nadav
Enter your E-mail address. We'll send you an e-mail with instructions to reset your password.