Hello All,
How does OpticStudio calculate polychromatic rms wavefront error? I tried my own formulation and couldn’t get a match.
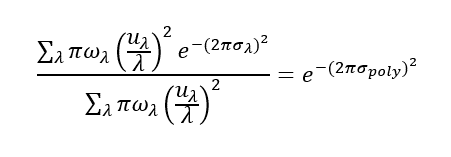
Derived from Born & Wolf, the sum of the Strehl weighted peak irradiances divided by same in absence of aberrations. u is the slope of the marginal ray or a/f in the Born & Wolf formulation.
Thanks, BJ Housand

