Skip to main content

Hi,



 



I was trying to select the lens from ThorLabs to collimate the diverging light to around 2 mm. But there seems to be a discrepancy between the size shown in the paraxial gaussian beam data and the semi-diameter of the image surface, which seems to automatically update according to the system design. I also tried to use the merit function GBPS to optimize the beam size, but the result does not seem to be correct according to the layout plot. Could someone explain what is the difference between the two sizes and which merit function to use in order to get the 2 mm collimated beam? Thank you!





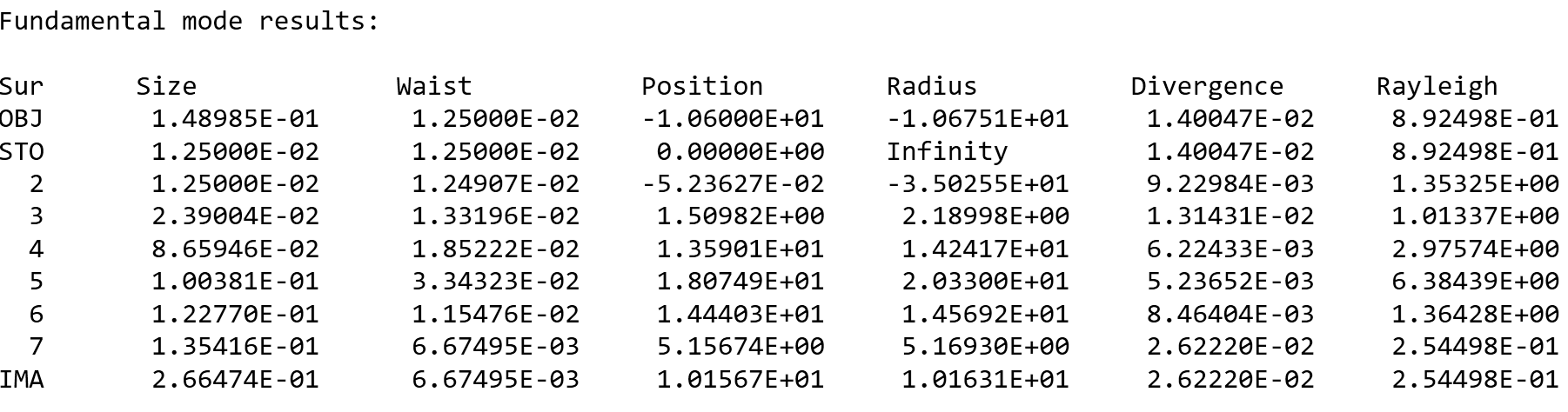
For this configuration, the paraxial gaussian beam data shows that the size of the beam at the image surface is 0.266 mm, which does not match the layout plot, where the semi-diameter of the lens is 3 mm. The size given by the semi-diameter of the image surface seems more reasonable.

Hello Haofeng,



I found two issues with your file. First, the thickness 10.6 mm on surface 0 occurs after the object, but since the beam waist is defined on surface 1, it occurs before the beam waist. Second, the waist size does not correspond to the 0.22 NA divergence in the ray trace. I corrected this with three changes:



Moved the 10.6 mm thickness to surface 1 with 0 thickness for surface 0.



Made surface 2 the stop so the entrance pupil is not located at the object.



Made the waist 0.000789265 mm so the beam divergence angle is equal to the 0.22 NA of the ray trace. (According to formula w0 = lambda / (Pi x Theta0).



With these changes the beam size at the image plane is 1.6 mm  semidiameter, which is pretty close to the ray trace.



I attach a ZAR file of the result.



Kind regards,



David



 



 



 

Thank you so much David, that solves the problem! But I am still wondering why there is still a difference between the semi-diameter of the image surface in LDE and the size of the beam at the image surface in the paraxial gaussian beam data?

Hi Haofeng,



The Paraxial Gaussian Beam Data analysis and the regular ray trace are going to trace through the system differently. The regular ray trace will propagate in a straight line and will not change direction until it comes into contact with another index of refraction. On the other hand, the Paraxial Gaussian Beam can diverge if it is outside the Rayleigh range. 



Another cause for the difference on the image plane is the regular ray trace starts from a point source which has no size. The paraxial Gaussian Beam must start having some size for the waist. 



-Kaleb 

Thank you Kaleb, that perfectly answers my question!

Reply


Community Posting GuidelinesCookie settings