Hi Tariq,

Not sure I understand the question. You should be able to change the distance in the Lens Data Editor, isn’t it your first surface thickness (assuming the telescope was modelled forward)?

Take care,

David

He's asking about what "resolution" his telescope will have at these different object distances.  The first thing to define is what is meant by "resolution".  How many pixels on target?  Dawes or Sparrow limit?  Smallest 3-bar group/element visible or imaged on a 1951 USAF target?  Lowest acceptable MTF value?  Resolution is a continuum rather than binary, so its meaning must first be stated.  Then we can help answer his question.

Oh, I see. Somehow, in my head the resolution was already established at 500m and infinity. Thus, it should be easy to get at other distances. Sorry for the confusion and thanks for the answer.

@Mike.Jones @David.Nguyen Thank you for your reply. Actually I am designing a camera lens which sensor specs are : size : 35.6×23.8 mm ; pixel pitch : 6 micron approx . I want to check the visibility of my camera until when the system can see a object (human, Drone) clearly.

For a well-corrected lens with negligible aberration, the resolution for imaging of distant objects (i.e., essentially infinite-conjugate imaging) is typically specified in terms of angular resolution.  Using the Rayleigh criterion for the minimum resolvable separation of two focused spots arising from two incoming plane waves (or at least approximate plane waves) associated with two points on a distant object, it is easy to show that the corresponding angular resolution depends only on wavelength and the lens diameter.  Here is what Warren Smith says (see Modern Optical Engineering -- I’ve attached a couple of relevant pages that have more detail):

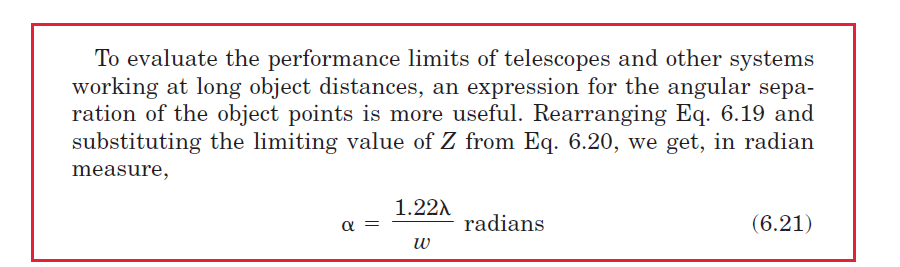
Here w is the lens diameter.  There is no dependence on the lens NA or its focal length.  This is why the James Webb telescope has a very large diameter (6.5 meters), but its NA is only 0.025.

So, if you take two fixed points on a distant object, they will have some angular separation as viewed from the lens.  If this angular separation is greater than alpha = 1.22(lambda/w), then the points are resolvable for an ideal lens.  Similarly, you can find the minimum resolvable separation of two points on a distant object by multiplying alpha (in radians) by the object distant.  As the object distance increases, this minimum resolvable object-space separation also increases, which generally means the object will appear more blurry.  Depending on what feature size on the object you are looking to resolve, you should be able to estimate the distance at which this feature is just resolvable -- for longer distances you won’t be able to resolve the feature, but for shorter distances the feature should only look better, assuming you optimize focus for any given object distance.

Of course, with an actual lens having aberration, ray tracing will tell you what the focused spot, or point spread function, looks like, and from the spot size you can estimate the corresponding angular resolution of the lens.  This assumes you are optics-limited; if the pixel size of your sensor limits resolution, then you need to estimate angular resolution by assuming an effective focused spot size (radius) of say ~2 pixels.  

Hope this helps…

Regards,

Jeff