Hi there friends from the OpticStudio community,
Today I have a question related to a general optics design problem.
I’m trying to generate a system similar to what is presented in the following publication:
https://opg.optica.org/ol/fulltext.cfm?uri=ol-42-6-1043&id=360492
In essence, the design problem consists of generating a high NA system which can be used to image molecules or atoms in a vacuum environment.
The approach used in this case consists of using a Weierstrass type solid immersion lens in combination with two aspherical surfaces in order to generate a collimated beam for an on axis point object.
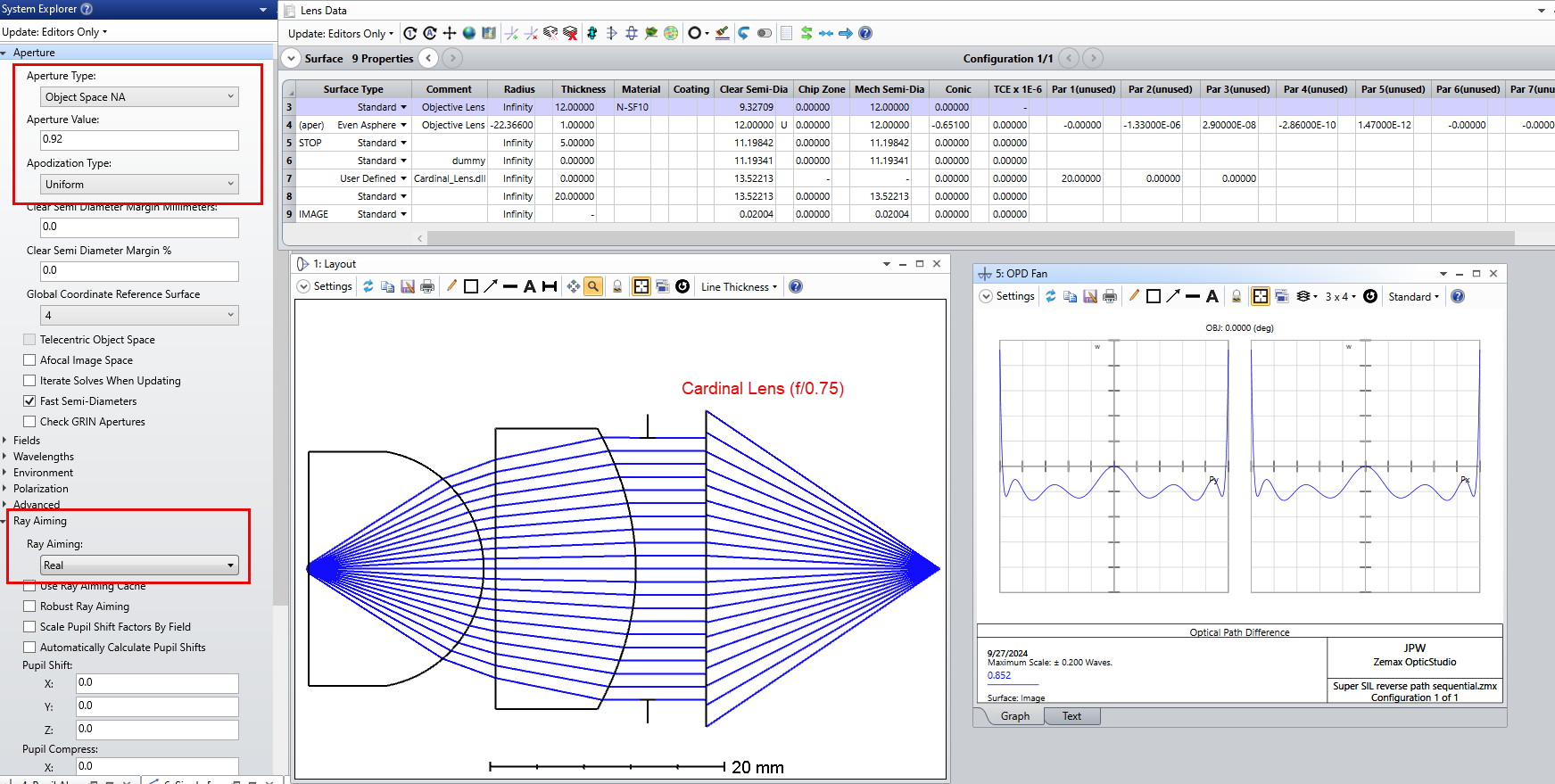
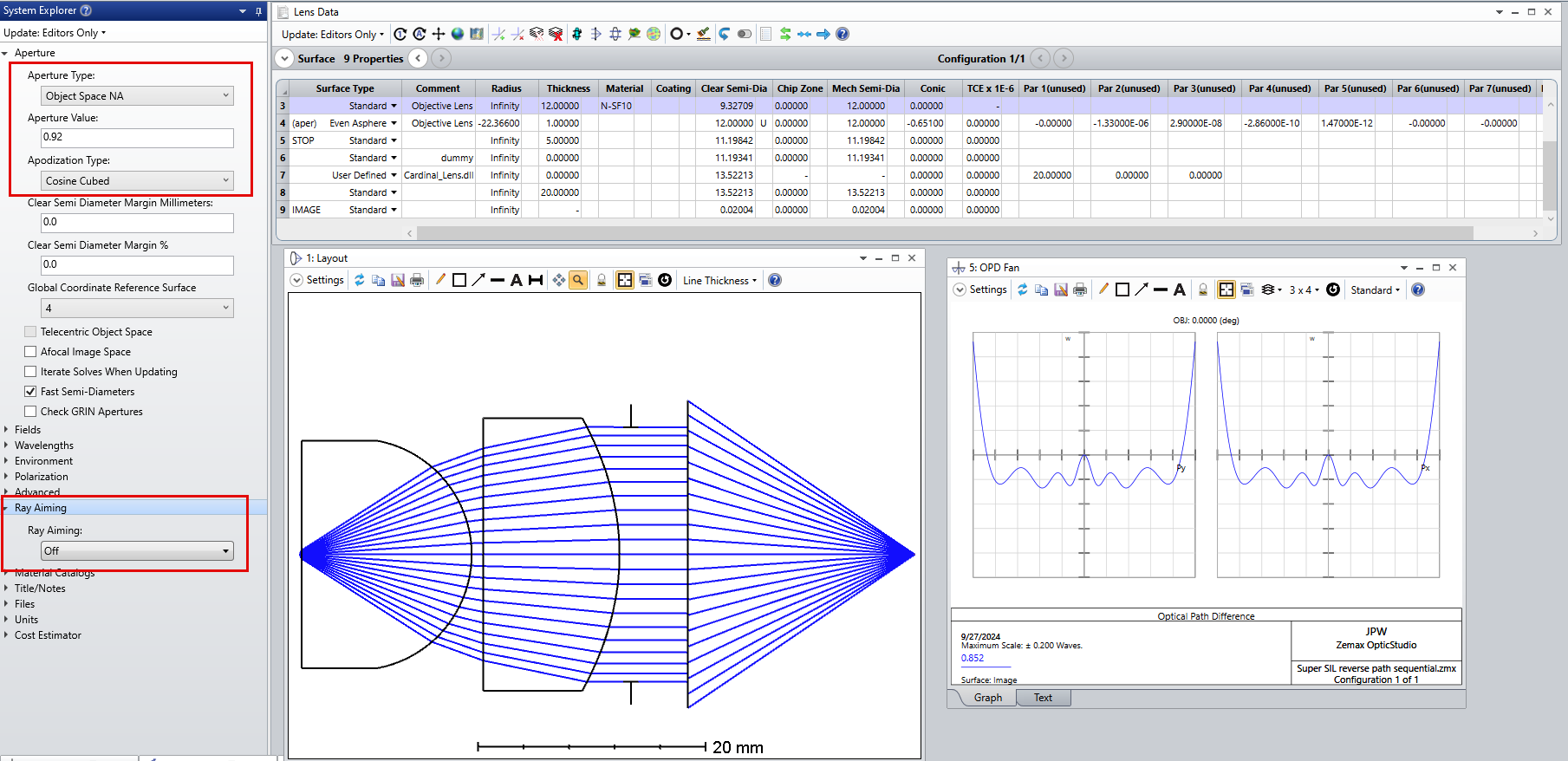
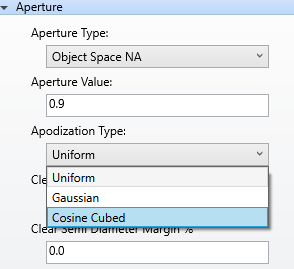
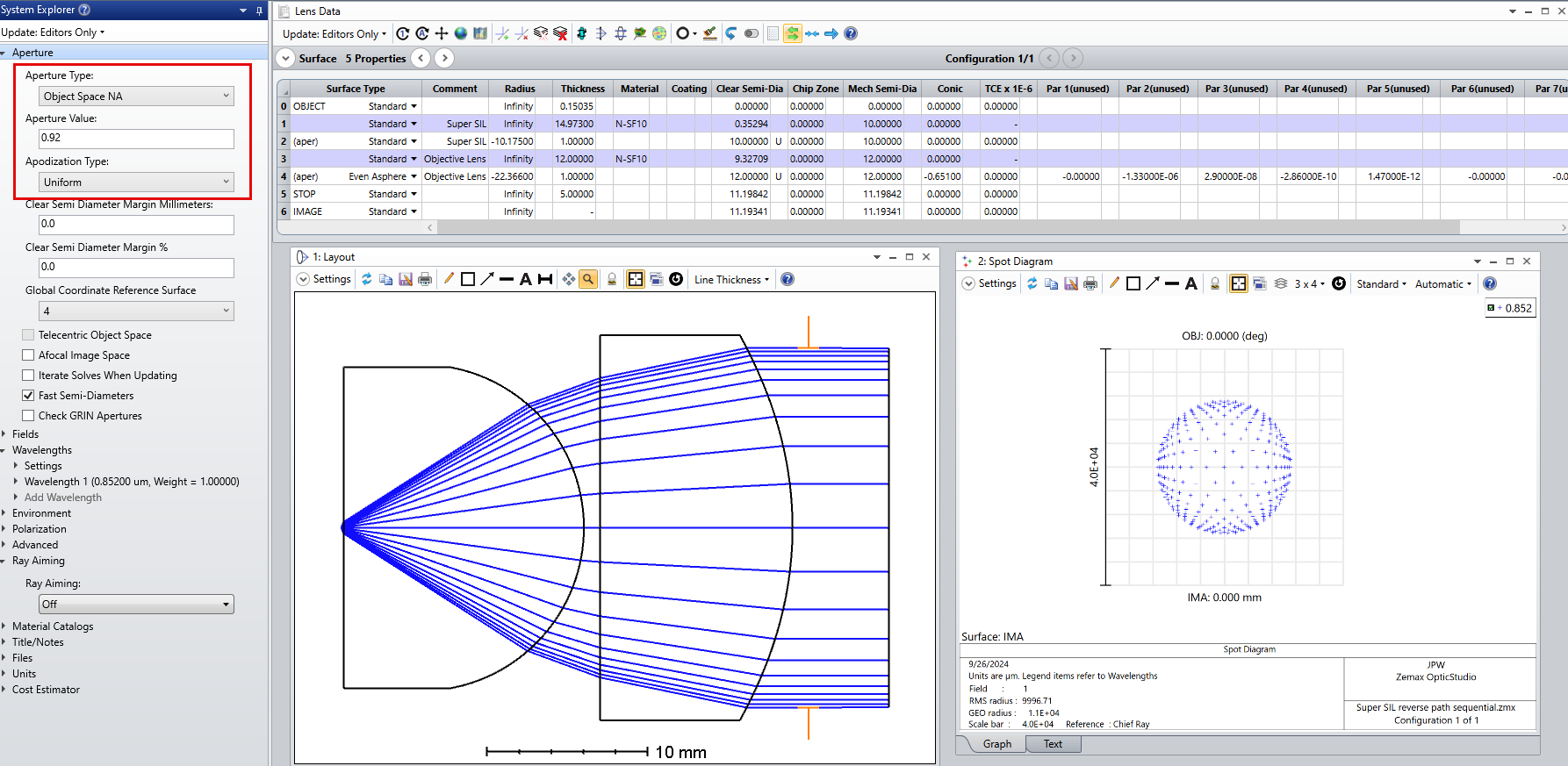
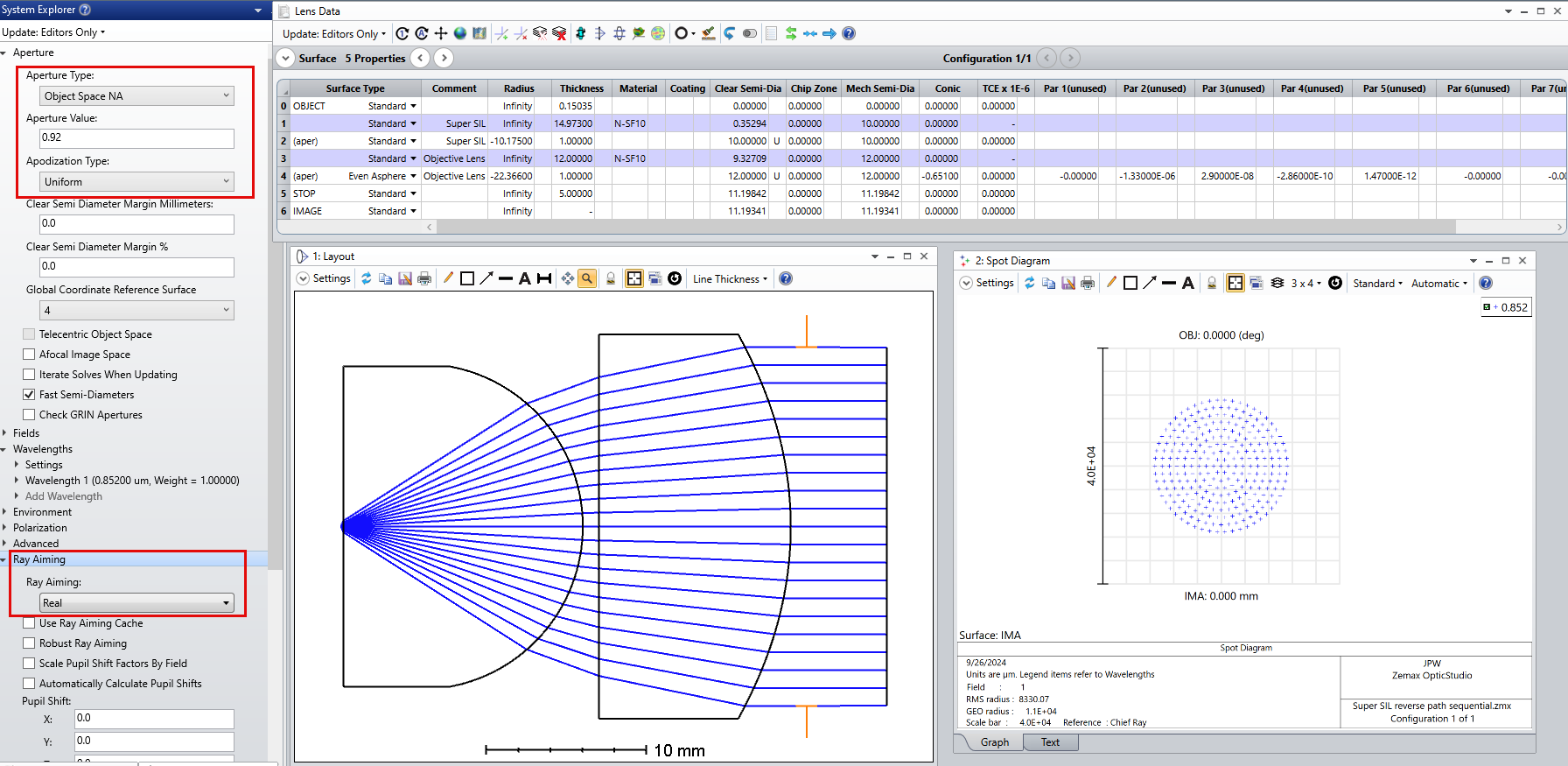
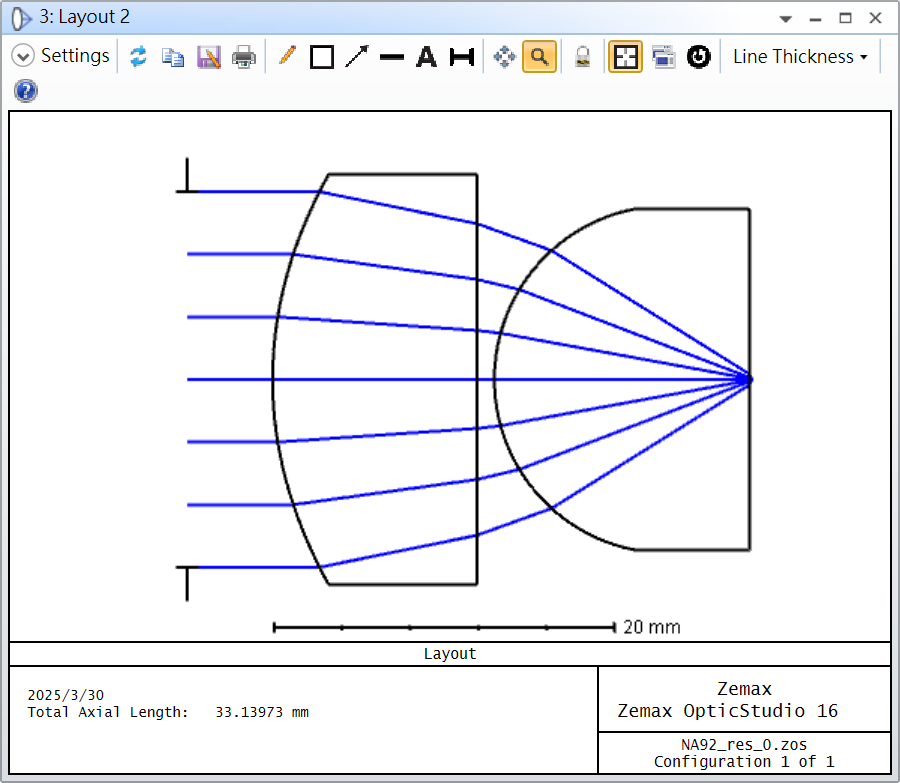
Since the geometry of the Weierstrass geometry is fixed, the only degrees of freedom that I have are the parameters related to my two aspherical surfaces. In the following figure you can take a look to the system I have at the moment:
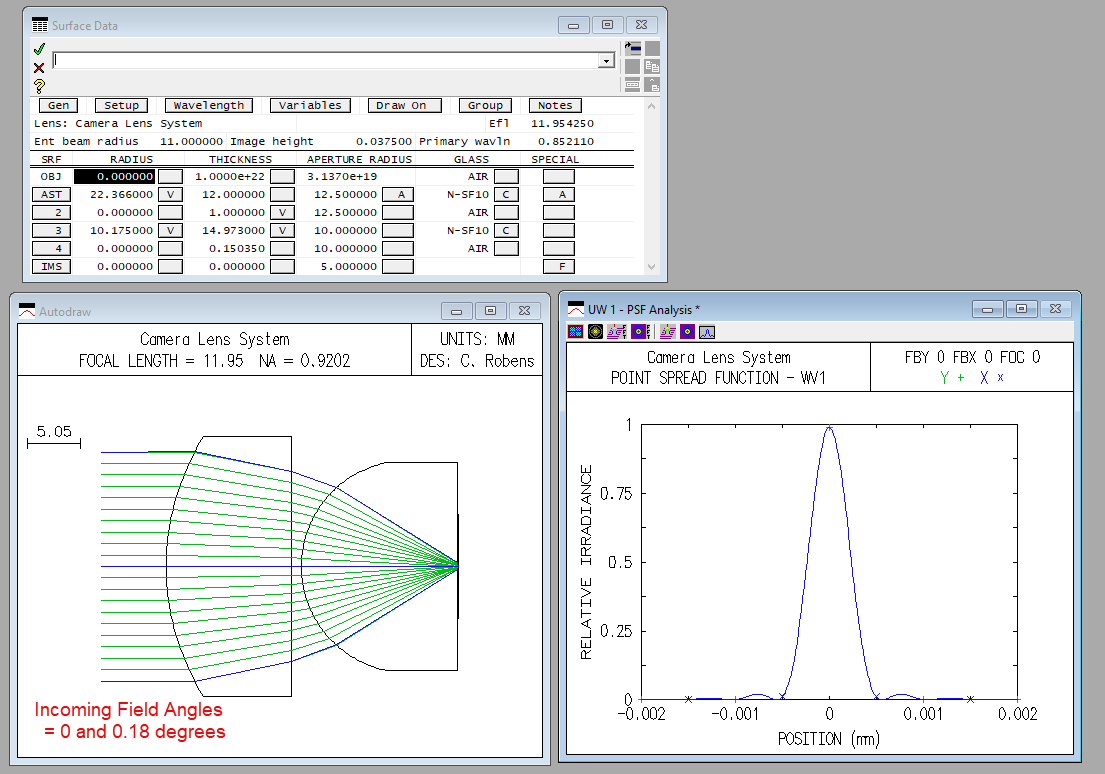
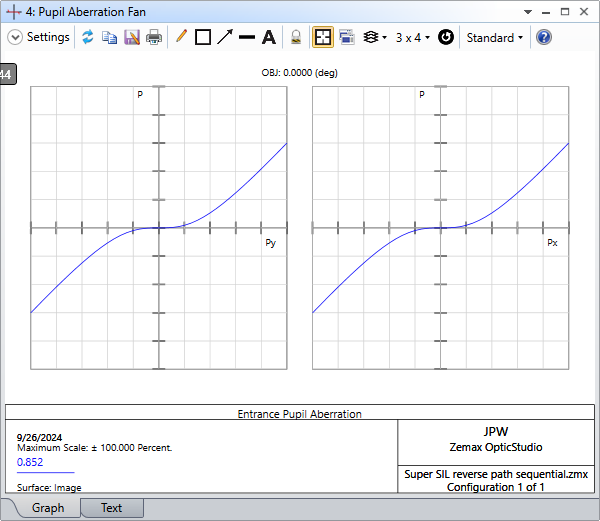
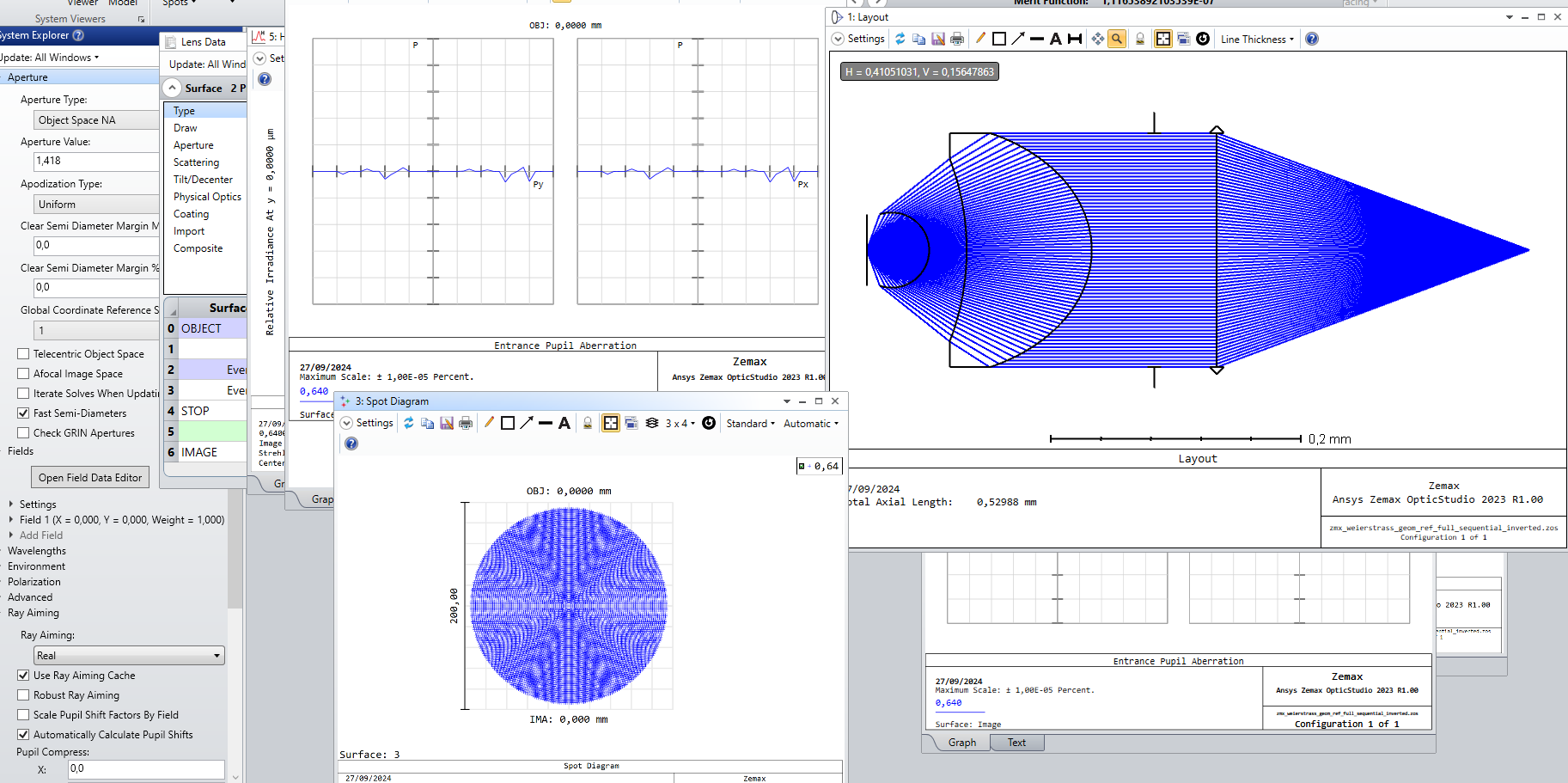
In this case, I optimized this system in the afocal configuration with the merit function defined to have a well corrected planar wavefront. After that I added the paraxial lens just to evaluat the PSF and to see how well corrected my system was.
From the PSF evaluation I get a Strehl ratio of 1.0 and also from the wavefront map I get a well corrected performance.
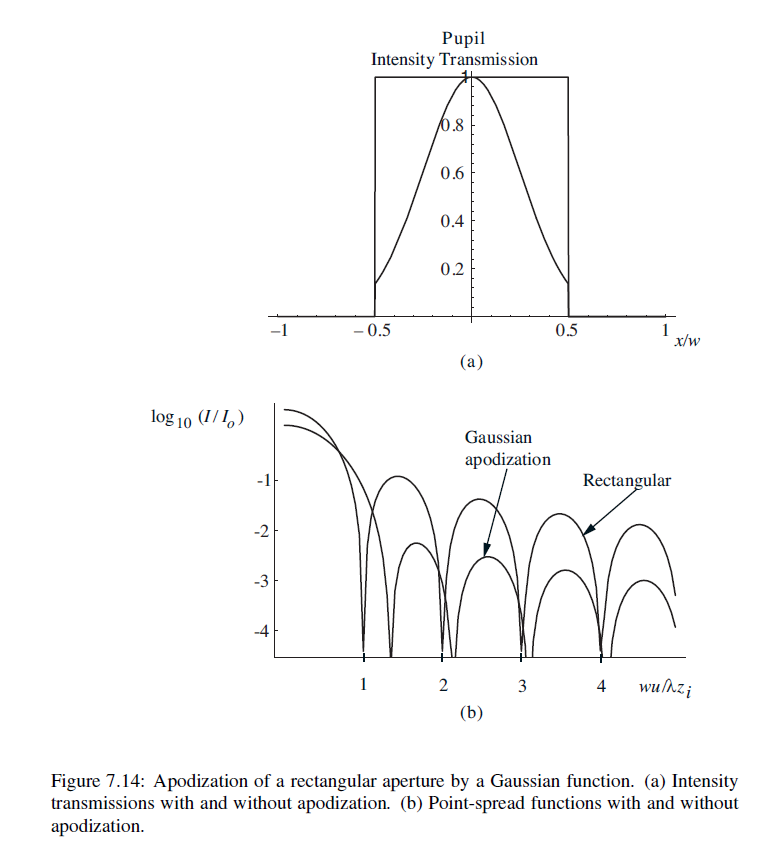
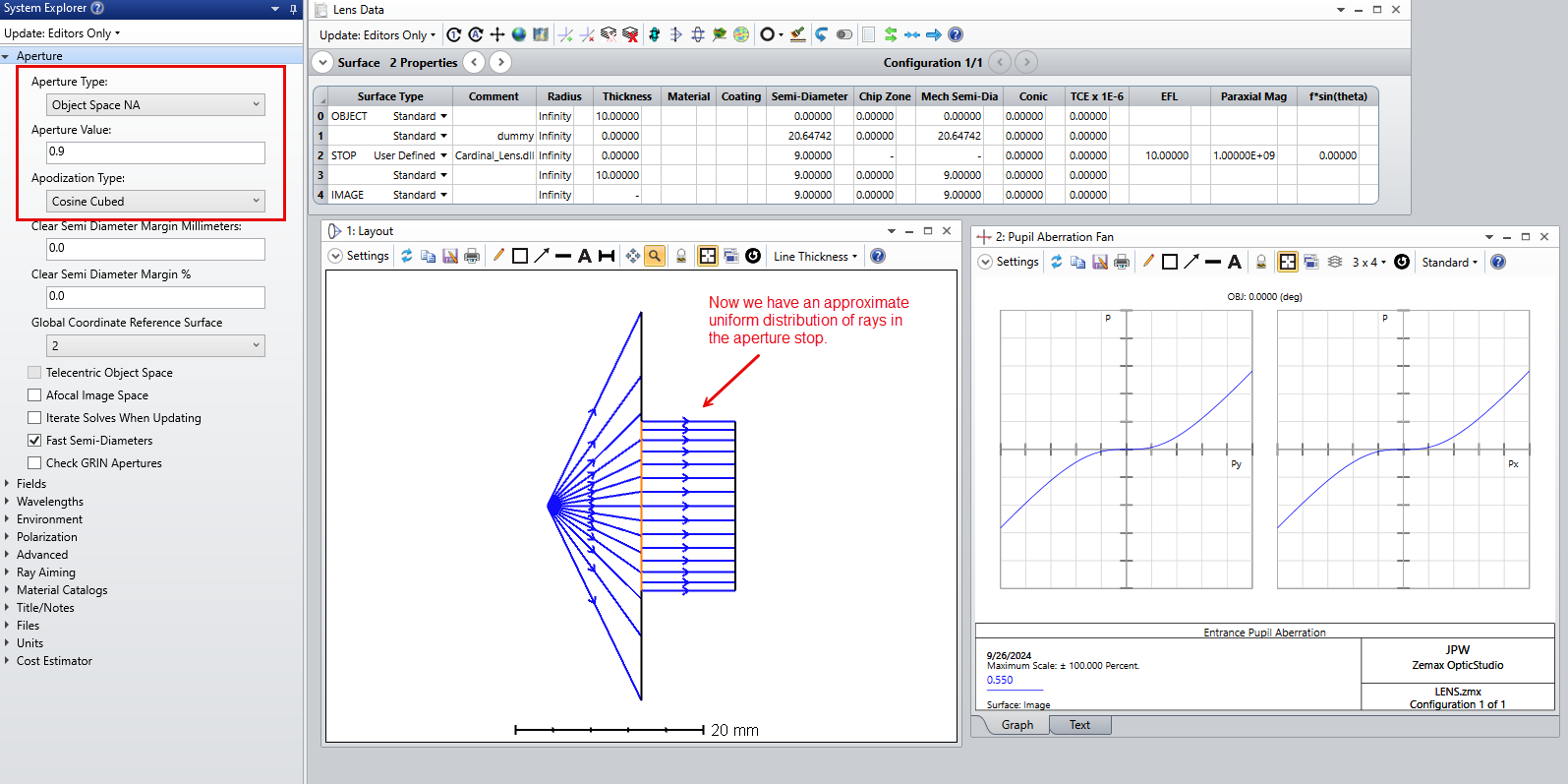
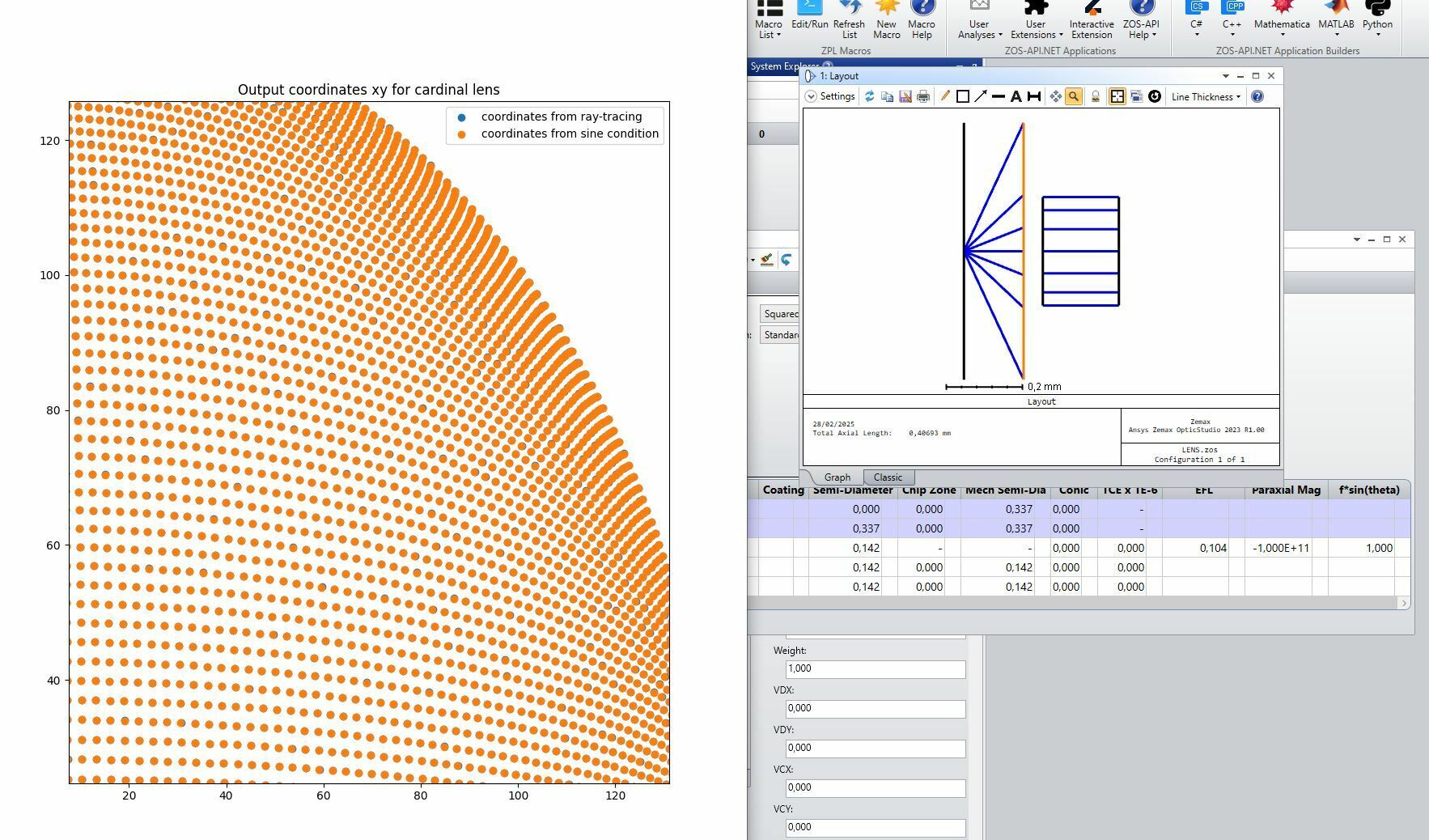
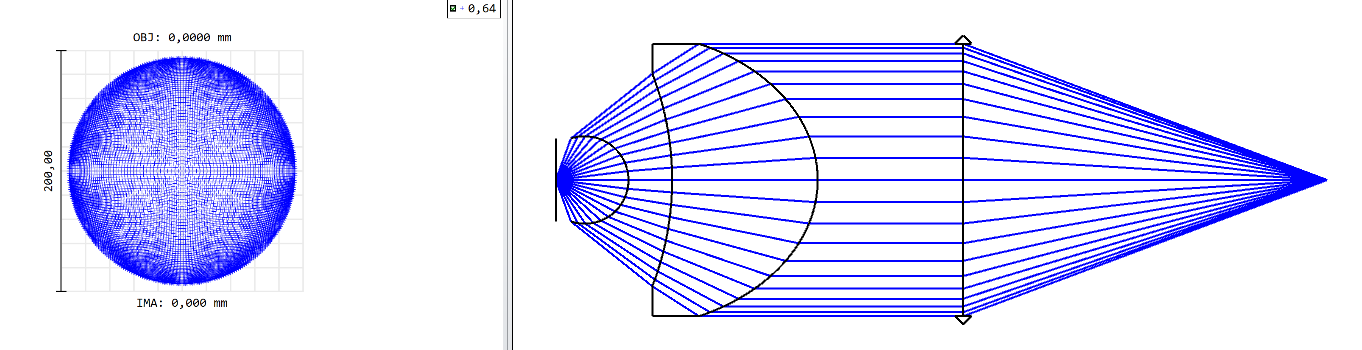
However, if you observe the ray density distribution right after the second aspherical surface and also from the spot diagram on the left side of the optical system, you can clearly see that the rays are not evenly distributed in the “collimated space”.
From what I know, if I had a uniform illumination right in front of the paraxial lens (given that the wavefront is well corrected) I would then get an ideal airy disk at the focal plane.
However, from such non-uniform ray density, I get an airy pattern that has stronger side lobes in contrast to the “ideal airy disk” case.
Having said this, my question is then the following: If I wanted to generate an ideal imaging system, should I also care for the ray density distribution in the collimated set of rays? Is this something that is considered while doing these type of optical designs? If yes, how can I impose this condition into the optimization routine?
All comments and feedback will be highly appreciated!